Fisika Study Center

This problem sample is taken from theoretical problems of first IphO that's conducted in Warsaw, Poland in 1967.

Problem 3
Consider two identical homogeneous balls, A and B, with the same initial temperatures. One of them is at rest on a horizontal plane, while the second one hangs on a thread (Fig. 6). The same quantities of heat have been supplied to both balls. Are the final temperatures of the balls the same or not? Justify your answer. (All kinds of heat losses are negligible.)

Fig. 6

Solution


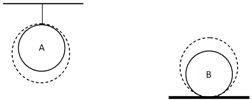
Fig. 7

As regards the text of the problem, the sentence “The same quantities of heat have been supplied to both balls.” is not too clear. We will follow intuitive understanding of this sentence, i.e. we will assume that both systems (A – the hanging ball and B – the ball resting on the plane) received the same portion of energy from outside. One should realize, however, that it is not the only possible interpretation. When the balls are warmed up, their mass centers are moving as the radii of the balls are changing. The mass center of the ball A goes down, while the mass center of the ball B goes up. It is shown in Fig. 7 (scale is not conserved). Displacement of the mass center corresponds to a change of the potential energy of the ball in the gravitational field. In case of the ball A the potential energy decreases. From the 1^st principle of thermodynamics it corresponds to additional heating of the ball. In case of the ball B the potential energy increases. From the 1^st principle of thermodynamics it corresponds to some “losses of the heat provided” for performing a mechanical work necessary to rise the ball. The net result is that the final temperature of the ball B should be lower than the final temperature of the ball A. The above effect is very small. For example, one may find (see later) that for balls made of lead, with radius 10 cm, and portion of heat equal to 50 kcal, the difference of the final temperatures of the balls is of order 10^-5 K. For spatial and time fluctuations such small quantity practically cannot be measured. Calculation of the difference of the final temperatures was not required from the participants. Nevertheless, we present it here as an element of discussion. We may assume that the work against the atmospheric pressure can be neglected. It is obvious that this work is small. Moreover, it is almost the same for both balls. So, it should not affect the difference of the temperatures substantially. We will assume that such quantities as specific heat of lead and coefficient of thermal expansion of lead are constant (i.e. do not depend on temperature). The heat used for changing the temperatures of balls may be written as

Q_i =mcΔt_i, where i = A or B,

Here: m denotes the mass of ball, c - the specific heat of lead and Δt_i - the change of the temperature of ball.

The changes of the potential energy of the balls are (neglecting signs):

ΔE_i =mgrαΔt_i, where i = A or B,

Here: g denotes the gravitational acceleration, r - initial radius of the ball, α - coefficient of thermal expansion of lead. We assume here that the thread does not change its length.

Taking into account conditions described in the text of the problem and the interpretation mentioned at the beginning of the solution, we may write:

Q = Q_A − AΔE_A, for the ball A,
Q = Q_B + AΔE_B, for the ball B.

A denotes the thermal equivalent of work: A ≈ 0.24 ^cal/_J. In fact, A is only a conversion ratio between calories and joules. If you use a system of units in which calories are not present, you may omit A at all.

Thus

Q = (mc − Amgrα)Δt_A, for the ball A,
Q = (mc + Amgrα)Δt_B, for the ball B,

and



Finally we get



(We neglected the term with α² as the coefficient α is very small.)

Now we may put the numerical values: Q = 50 kcal, A ≈ 0.24 cal/J, g ≈ 9.8 m/s², m ≈ 47 kg (mass of the lead ball with radius equal to 10 cm), r = 0.1 m, c ≈ 0.031 cal/(g⋅K), α ≈ 29⋅10^-6 K^-1. After calculations we get Δt ≈ 1.5 ⋅ 10^-5 K.

Sources/Literatures :
-Waldemar Gorzkowski, Institute of Physics, Polish Academy of Sciences, Warsaw, Poland
-The Olympic home page www.jyu.fi/ipho